When you were studying animation, you probably thought you had no need for math. But one thing you definitely need to know about is the value known as pi, and how you can use pi in animation.
Why pi?
For centuries, mathematicians were obsessed with the relationship between the diameter of a circle and its circumference (the distance around it). They knew there had to be an exact formula; it just took a while to find it. When they figured it out, they named it pi after the Greek word for the letter P, and it opened up a whole new world for geometry.
It also opens up a world for animators. There are a number of scenarios where you’re going to need to know about pi.
What is pi, exactly?
Pi is always the same number: 3.14. Actually, it’s 3.14159 and then an infinite series of numbers. But for most applications, 3.14 works just fine.
The circumference of a circle is always its diameter times pi.
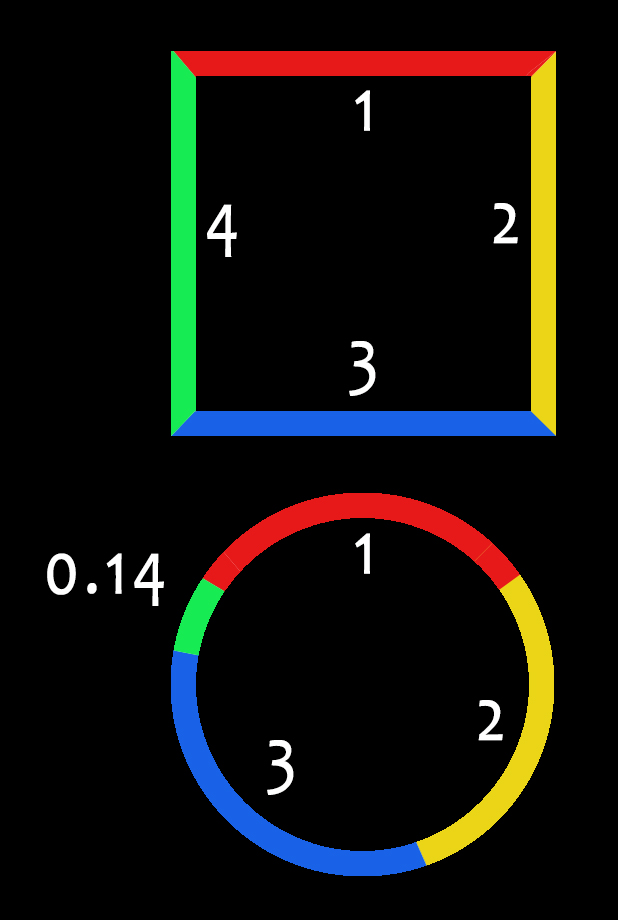
To understand pi, consider a square with sides that are 1 unit long. It could be one inch or one meter, it’s all the same. You can see the relationship between the circumference of the square and the circle. For the square, it’s 4, but for the circle it’s 3.14.
If you look at the diagram, you’ll see that the diameter of the circle is the same as one side of the square. So instead of one side of the square going around the circle 4 times, it goes around 3.14 times. This is true no matter the size of the circle.
You’ll need these concepts when calculating things like how many times the wheels will turn when a car is moving. If you’re thinking of getting into scripting for animation, you’ll need to use pi for all manner of calculations.